Non Verbal Reasoning Questions and Answers
Select the alternative which represents three out of the five alternative figures which when fitted into each other would form a complete square.
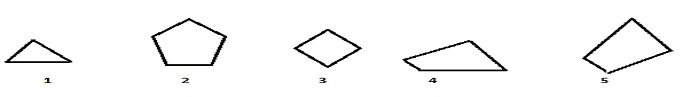
ANSWER: 145
Select the alternative which represents three out of the five alternative figures which when fitted into each other would form a complete square.
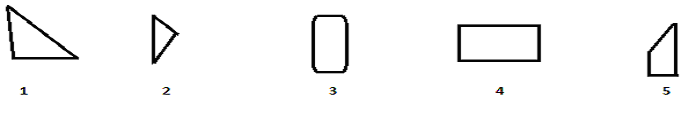
ANSWER: None of these
Find out which of the figure (a), (b), (c), (d) can be formed from the pieces given in fig. (X).
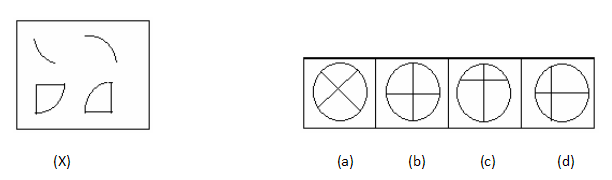
ANSWER: (b)
Find out which of the figure (a), (b), (c), (d) can be formed from the pieces given in fig. (X).
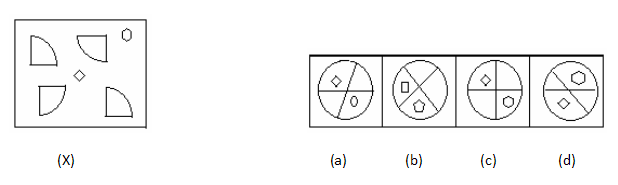
ANSWER: (c)
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have no face painted?
Cubes have no face painted = Inner Cubes (No Colour): We can find out the total number of cubes without any colour on any side (inner cube) with this formula: (X-2)^3
Implementation of formula: X = 4
(4-2)^3 = 2^3 = 8
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have only one face painted?
Cubes have only one face painted = Central cubes : In middle of faces & has only one coloured side.
We can find out the total number of cubes with singe colour on any side with this formula: 6(X-2)^2
Implementation of formula: X = 4
6(4-2)^2 = 6(2)^2 = 24
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have only two faces painted?
Cubes have only two faces painted = Middle Cubes: In middle of edges and have two coloured sides.
We can find out the total number of cubes with singe colour on any side with this formula: 12(X-2)
Implementation of formula: X = 4
12(4-2) = 12(2) = 24
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have only three faces painted?
Cubes have only three faces painted = Corner cubes : Cubes on corners and have three coloured sides.
A cube can have only 8 cut-corner cubes with colours on three sides. Hence answer will be always the same = 8.
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have three faces painted with different colours?
Cubes have three faces painted = Corner cubes : Cubes on corners and have three coloured sides.
A cube can have only 8 cut-corner cubes with colours on three sides. Hence answer will be always the same = 8.
A solid cube of each side 8 cm, has been painted red, blue and black on pairs of opposite faces. It is then cut into cubical blocks of each side 2 cm.
How many cubes have two faces painted red and black and all other faces unpainted?
Cubes have two faces painted red and black and all other faces unpainted = 2+2 = 4